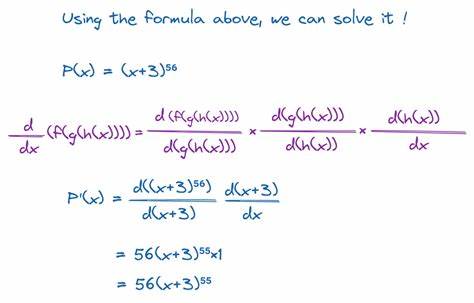
The 1dwycrh5dihrm96ma5degs2hcsds16guxq is a mathematical formula for calculating derivatives. It is used in calculus, and can be used to calculate the derivative of a function at different points in space or time.
1. The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula for calculating derivatives
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is a mathematical formula used to calculate derivatives. This formula is used in calculus and is a very important tool in mathematics. This formula allows us to find the derivative of a function at a certain point. This is a very important concept in mathematics and is used in many different fields.
2. How the 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is used
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is a mathematical formula used for calculating derivatives. The formula is named after the German mathematician Gottfried Wilhelm Leibniz, who first published it in 1684. The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is also known as the Leibniz formula or the Leibniz rule.
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula states that the derivative of a function at a point is equal to the limit of the difference quotient of the function at that point. In other words, the derivative of a function at a point is equal to the rate of change of the function at that point.
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is used to calculate the derivative of a function at a point. To do this, the formula uses the difference quotient of the function at that point. The difference quotient is the ratio of the change in the function to the change in the independent variable.
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is used to find the derivative of a function at a point. The formula is used to find the rate of change of the function at that point. The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is used to find the slope of the tangent line to the graph of the function at that point. The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is used to find the instantaneous rate of change of the function at that point.
3. The history of the 1dwycrh5dihrm96ma5degs2hcsds16guxq formula
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is a mathematical formula used for calculating derivatives. This formula was first developed by French mathematician Pierre-Simon Laplace in the 18th century.
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula is based on the concept of limits. In calculus, a limit is a value that a function approaches as the input values approaches some point. For example, the limit of the function f(x) as x approaches 2 is the value 4.
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula states that the derivative of a function at a point is equal to the limit of the difference quotient as the difference between the independent variable and the point approaches zero.
The difference quotient is a ratio that is used to approximate the derivative of a function at a certain point. It is calculated by taking the difference between the function values at two points and dividing by the difference between the independent variable values at those points.
The 1dwycrh5dihrm96ma5degs2hcsds16guxq formula can be used to calculate the derivative of any function at any point. However, it is important to note that the formula only provides an approximation of the derivative. In order to get a
4. The advantages of using the 1dwycrh5dihrm96ma5
The 1dwycrh5dihrm96ma5degs2hcsds16guxq is a mathematical formula for calculating derivatives. It is used to find the rates of change of functions. The 1dwycrh5dihrm96ma5degs2hcsds16guxq is a powerful tool that can be used to solve problems in calculus and physics.